Scale of the Day: E Flat Mixolydian no 4, diminished 5 mapped to the Square-root-of-2

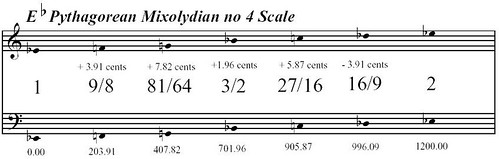
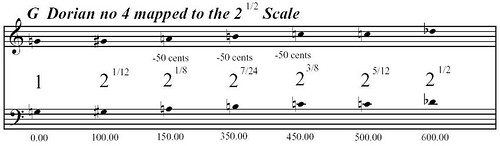
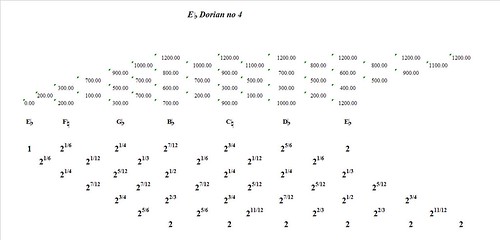
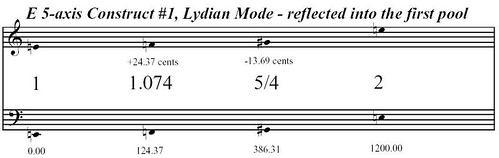
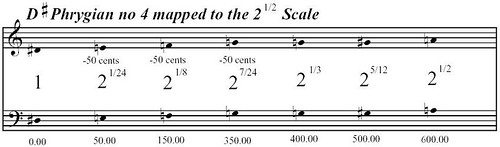
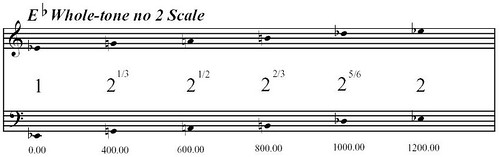
The E Flat Mixolydian no 4, diminished 5 mapped to the Square-root-of-2 Scale. With 6 notes spread out over 600 cents - with just one quarter-tone - this scale poses an interesting cognitive challenge. Square-root-of-2 based scales already pose the challenge of treating the 600-cent so-called "tritone" as a pitch class equivalent. Which is difficult to convey sonically since the "double tritone" is the familiar 1200-cent octave that is hard-wired in the human auditory system as strongly equivalent. In practice, the 1200-cent interval will always seem more "equivalent" than the 600-cent or even 1800-cent interval. So this particular scale will sound like a standard chromatic scale with two quarter-tones as this 6-tone sequence repeats at each tritone. The conceptual challenge is to compositionally treat 600-cent intervals as pitch class equivalents and allow the subtle contour of the single quarter-tone to emerge from within the harmonic fabric.

Scale of the Day: E Flat Pythagorean Mixolydian no 4

The E Flat Pythagorean Mixolydian no 4 Scale. This is the 3-limit (Pythagorean) just intonation version of this subtractive scale. The 32/27 minor third appears between the 81/64 and 3/2 where the missing 4/3 perfect fourth would have been. Without that fourth degree the 16/9 becomes the only utonal member of this particular set - a property normally associated with Ionian scales. But the 1024/729 diminished fifth between the 81/64 and the 16/9 give this scale its unmistakable Mixolydian flavor.

Scale of the Day: G Dorian no 4 mapped to the Square-root-of-2

The G Dorian mapped to the Square-root-of-2 Scale. The intervallic symmetry of the Dorian Scale with the gap of the omitted fourth degree shrunk down to a quarter-tone scale.

Scale of the Day: E Flat Dorian no 4

The intervallic content of the E Flat Dorian no 4 Scale. The missing fourth degree breaks up the symmetry of the Dorian scale and leaves the perfect fifth without its counterbalancing inversion.
Pardon the brief interruption in posting as I make may way to a new time zone.

Scale of the Day: G Dorian no 4, diminished 5

The G Dorian no 4, diminished 5 Scale as one would find it on any conventionally tuned, equal tempered instrument. The diminished 5 helps closed the gap opened up by the missing fourth degree and restores some of the intervallic symmetry associated with dorian scales that is lost with that gap. The 300 cent minor third/augmented second that opens up on either side of that diminished fifth makes this one harmonically interesting.

Scale of the Day: C Sharp Aeolian no 4

The C Sharp Aeolian no 4 Scale as one would find it on any conventionally tuned, equal tempered instrument.

Posting is going to be sporadic for the next little while. There are some major upheavals underway in the world of HurdAudio. Look for a major update on the blogger profile in the next few days...

Scale of the Day: E 5-axis, Construct #1 - Lydian Mode - reflected into the first pool

The E 5-axis, Construct #1 - Lydian Mode - reflected into the first pool Scale. This scale is more conceptually interesting than practical. This isn't exactly the kind of scale one would "substitute" in everyday applications. With just three notes spanning an octave it is harmonically impoverished - which becomes the sonic focus and minimalist challenge when approaching it compositionally. First, one divides the octave with a 5/4 just major third. Then one applies that same proportions to divide the 5/4 interval when "reflecting" the relative intervallic size within the interval between the tonic and the 5/4. The 1.074 being the same relative size to the 5/4 as the 5/4 is to the 2.

Scale of the Day: D Sharp Phrygian no 4 mapped to the Square-root-of-2

The D Sharp Phrygian no 4 mapped to the Square-root-of-2 Scale. A six-note scale spanning the 600-cent equal tempered tritone featuring three quarter-tones and a chasm just 200 cents wide where the missing "fourth" degree would be. The contrast of the soft dissonance of that 200 cent interval between the 3rd and 4th degree with the hard dissonance of the 50 cents separating the 4th and 5th degree gives this scale its color.

Scale of the Day: D Sharp Phrygian no 4, major 6

The D Sharp Phrygian no 4, major 6 Scale as one would find it on any conventionally tuned, equal tempered instrument. This altered, subtractive version of the Phrygian scale retains its "Phrygian darkness" with the tritone between the minor second and perfect fifth while introducing a "Dorian brightness" with the tritone found between the minor third and major sixth scale degrees. The missing fourth degree adds a nice gap that spans a major third between adjacent scale members right in the middle of the scale.

Scale of the Day: A Sharp Locrian no 4

The A Sharp Locrian no 4 Scale as one would find it on any conventionally tuned, equal tempered instrument.

Scale of the Day: E Flat Whole-tone no 2

The E Flat Whole-tone no 2 Scale as one would find it on any conventionally tuned, equal tempered instrument. This is the first pentatonic scale in the particular sequence of scales that I'm working through. Which I find odd since I find pentatonic scales so interesting to work with compositionally. This particular scale is a whole-tone minus the second scale degree. So it is a pentatonic by subtraction. And like the whole-tone scale it is a "soft" scale with no dissonance stronger than the "tritone" or major second/minor seventh. To my ears, those 'dissonances' don't exactly offset the strong consonance of the major thirds/minor sixths. But the gap opened up by eliminating the second degree gives a noticeable cluster leading up to the tonic.