(Part 2 in a continuing series of posts regarding melodic manipulation and development) Let's turn our attention to a second melodic line from an early composition of mine:


example 1: Melody #2
Transposition
Transposition is one of the simpler - more immediate melodic manipulations. Each pitch-class is either raised or lowered by a single fixed interval. For example, here is Melody #2 raised by a minor third:

example 2: Melody #2 Transposed by a minor third
Just Intonation Strategies for Non-tonal Melodic Lines
Aesthetically, non-tonal melodies are well suited for a free style approach to intonation as articulated by Lou Harrison. In this manner it is possible for a composition to roam freely in tuning space without being bound by a fixed scale. And as non-tonal melodies are already unbound by a fixed "tonic" or scale structure to begin with they make a great candidate for free style intonation strategies. There are a couple of different ways of going about this.
The first approach is to tune each note relative to the note that preceded it. So a possible 5-limit realization of this melody might look like this:

The frequency ratios quickly make use of extremely large integers as this melodic fragment becomes unhinged from any sort of tonal center or scale reference. The linear tuning logic from note to note is actually fairly simple even as things move quickly away from the original E Flat (1). The C natural comes in as a 5/3 major sixth relative to E Flat. The augmented fourth that follows is a 45/32 augmented fourth relative to the 5/3 (with frequency ratios, one adds intervals by multiplying, therefore, (5/3)(45/32) = (75/64)). The 25/16 is a 4/3 perfect fourth relative to the 75/64. And so on until the E Flat "returns" as a 3805/32768 after cycling through so many intervals. Sonically, this is harmonically intelligible and unsettling at the same time.

example 3: Melody #2 In 5-limit free style
The frequency ratios quickly make use of extremely large integers as this melodic fragment becomes unhinged from any sort of tonal center or scale reference. The linear tuning logic from note to note is actually fairly simple even as things move quickly away from the original E Flat (1). The C natural comes in as a 5/3 major sixth relative to E Flat. The augmented fourth that follows is a 45/32 augmented fourth relative to the 5/3 (with frequency ratios, one adds intervals by multiplying, therefore, (5/3)(45/32) = (75/64)). The 25/16 is a 4/3 perfect fourth relative to the 75/64. And so on until the E Flat "returns" as a 3805/32768 after cycling through so many intervals. Sonically, this is harmonically intelligible and unsettling at the same time.
The second approach involves isolating phrases and tuning each note relative to a significant pitch found within each phrase. Using this approach, an alternate 5-limit realization of this melody might look like this:
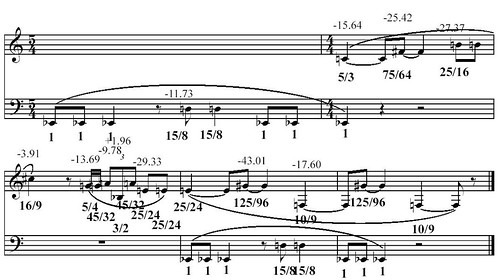
In this example the phrasing marks are used to delineate discrete gestalt units (as opposed to performance indicators). The first note of each "phrase" unit, or gestalt, is tuned relative to the first note of the gestalt that preceded it. Each subsequent note is tuned relative to the first note of the gestalt. In this manner the rate of travel through tuning space is slowed down considerably compared to example 3 even though this technique generally amplifies the "unhinged" qualities of non-tonal melody with such a liquid approach to intonation.
5-limit just intonation is best suited for triadic, diatonic, tonal music. A non-tonal melody such as this one is not a particularly good fit for traditional 5-limit just intonation. The 5-limit system features good major thirds/minor sixths (produced by the prime factor 5) and perfect fifths/perfect fourths (produced by the prime factor 3) prominently. Meloldy #2 makes prominent use of major 7ths and augmented 4ths. So this suggests a system built on prime factors that represent these intervals. One could "swap" out the 5 and 3 of the 2-dimensional tuning lattice with a 31 (for the 31/16 major seventh) and 11 (for the 11/8 augmented fourth). So, melody #2 using a just intonation system limited to the prime factors of 2 (the octave), 11 and 31 might look like this:

Now this melody begins to take on an inner logic as it carves out a distinct, and unfamiliar harmonic vocabulary. This could easily spin outward from here into a full composition that explores the sonic qualities of an 11, 31-space.
Other possible strategies for applying just-intonation to non-tonal melodies include:
I'll need to flesh these out in a future posting.

example 4: Melody #2 In 5-limit free style, using phrase approach
In this example the phrasing marks are used to delineate discrete gestalt units (as opposed to performance indicators). The first note of each "phrase" unit, or gestalt, is tuned relative to the first note of the gestalt that preceded it. Each subsequent note is tuned relative to the first note of the gestalt. In this manner the rate of travel through tuning space is slowed down considerably compared to example 3 even though this technique generally amplifies the "unhinged" qualities of non-tonal melody with such a liquid approach to intonation.
5-limit just intonation is best suited for triadic, diatonic, tonal music. A non-tonal melody such as this one is not a particularly good fit for traditional 5-limit just intonation. The 5-limit system features good major thirds/minor sixths (produced by the prime factor 5) and perfect fifths/perfect fourths (produced by the prime factor 3) prominently. Meloldy #2 makes prominent use of major 7ths and augmented 4ths. So this suggests a system built on prime factors that represent these intervals. One could "swap" out the 5 and 3 of the 2-dimensional tuning lattice with a 31 (for the 31/16 major seventh) and 11 (for the 11/8 augmented fourth). So, melody #2 using a just intonation system limited to the prime factors of 2 (the octave), 11 and 31 might look like this:

example 5: Melody #2 In 11, 31-just intonation
Now this melody begins to take on an inner logic as it carves out a distinct, and unfamiliar harmonic vocabulary. This could easily spin outward from here into a full composition that explores the sonic qualities of an 11, 31-space.
Other possible strategies for applying just-intonation to non-tonal melodies include:
- 3 or greater dimensional lattice spaces.
- Single dimension systems (such as a Pythagorean system).
- An unlimited, multi-dimensional lattice system that uses single primes for each interval type.
- Cyclical "1/1" values assigned to a new member each time through a tone-row.
I'll need to flesh these out in a future posting.


No comments:
Post a Comment